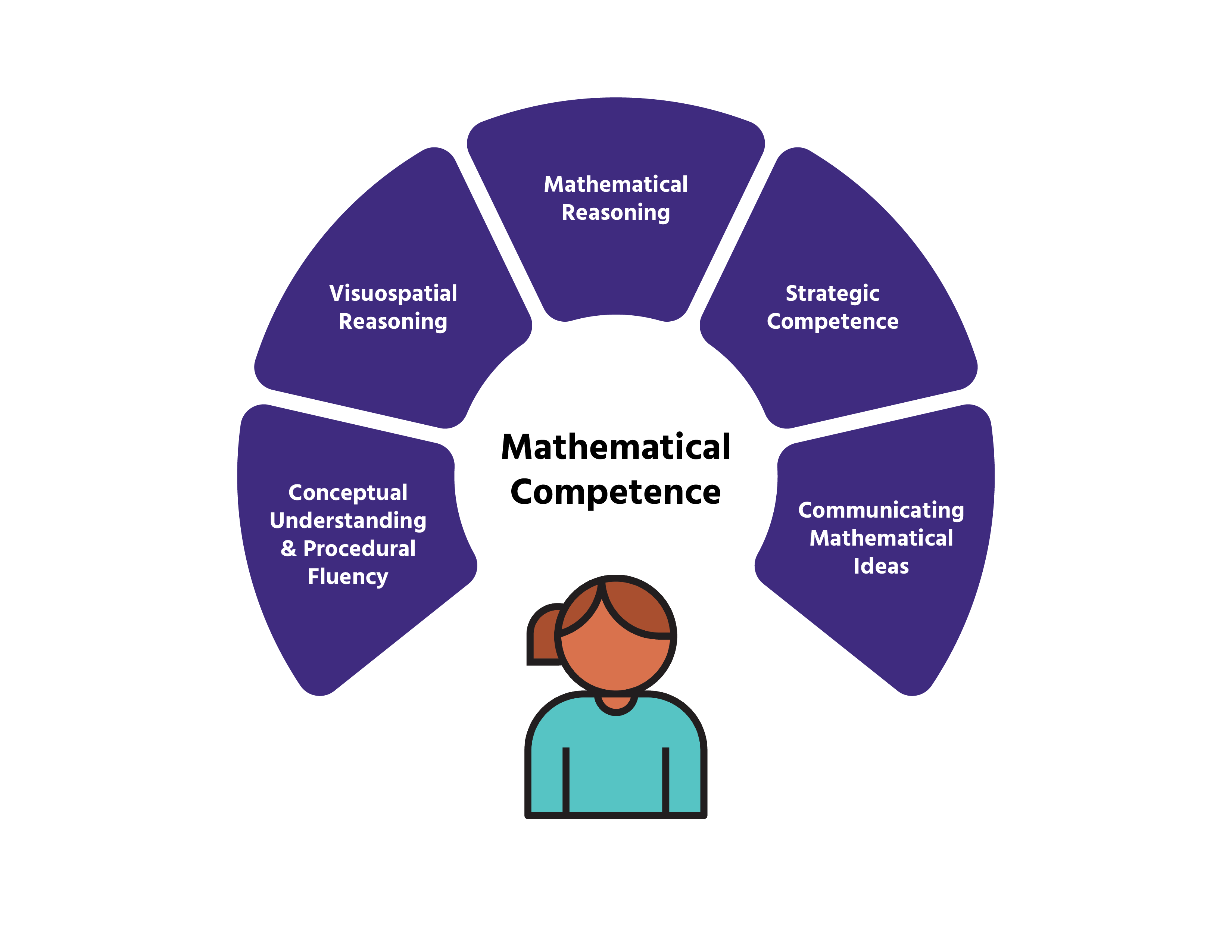
Brain research tells us that providing opportunities for students with these levers of mathematical competence can create the conditions for high-quality math instruction and an active learning environment. We explore mathematical competence more throughout this blog. 

Unlocking Mathematical Potential: From Brain Science to Effective Strategies

Moving beyond “what” students learn, mathematical competence hinges on understanding “why” and “how.” Brain research sheds light on this complex process, revealing how interconnected networks support the growth of mathematical knowledge and skills. 

The Visuospatial Network plays a crucial role in visualizing and manipulating abstract symbols and relationships in math. This network is active when we mentally rotate shapes, imagine fractions on a number line, or track spatial relationships between numbers. 

The Semantic Network is responsible for understanding the meaning behind mathematical symbols and equations. This network helps us connect new concepts to existing knowledge, choose the appropriate representations and strategies for solving problems, and apply mathematical principles in real-world situations. 

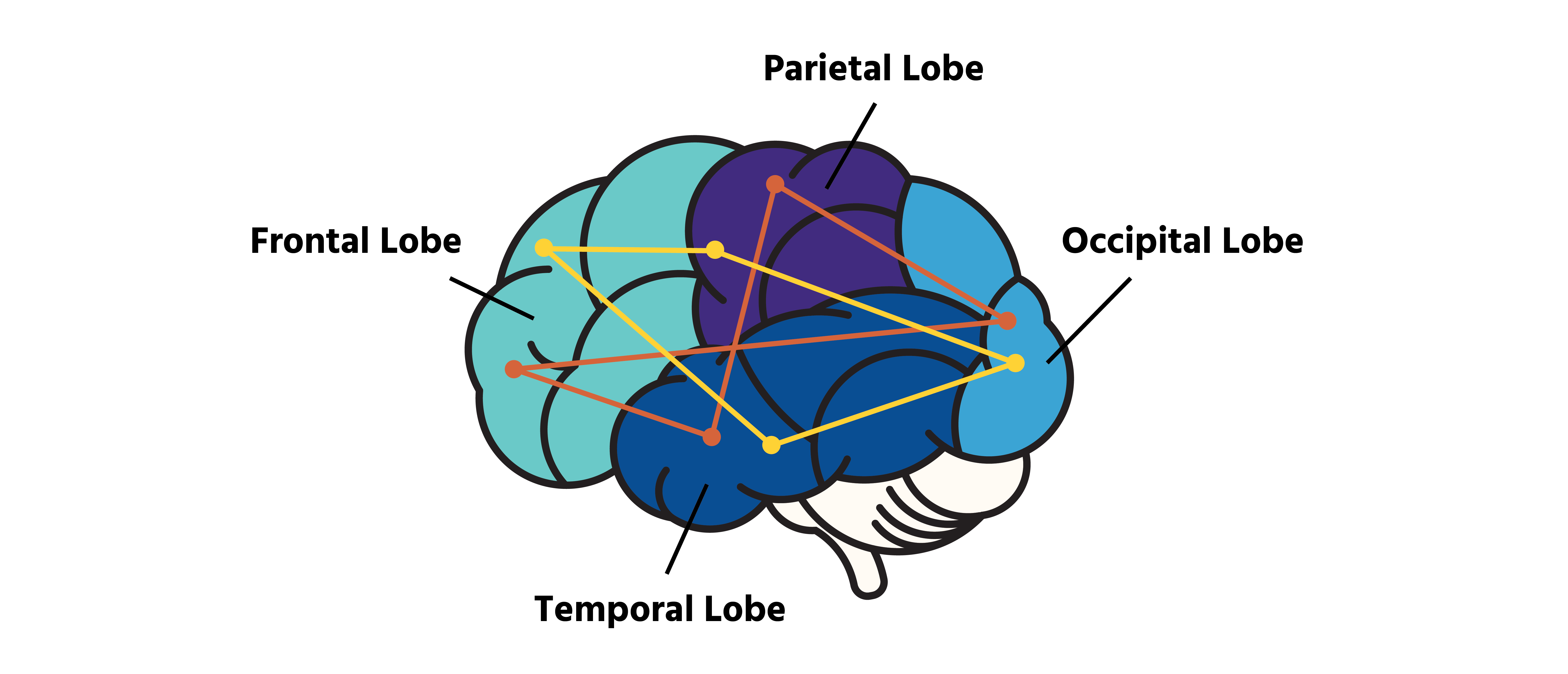
These networks, while impressive, merely hint at the vast orchestra of abilities conducted by the human brain. The brain’s remarkable flexibility in learning, representing, abstracting, and making connections lays the groundwork for sophisticated cognitive skills like critical thinking and problem-solving. By actively analyzing, synthesizing, and generalizing information, we become efficient problem solvers who can apply knowledge to new situations, and navigate uncertainty and complex problems with confidence and creativity.

Tips for Developing Mathematical Competence with Students

Ready to unlock your students’ mathematical potential? In this section, we explore practical techniques informed by how the brain learns math to supercharge your classroom.

Conceptual Understanding & Procedural Fluency: Break it Down, Build it Up

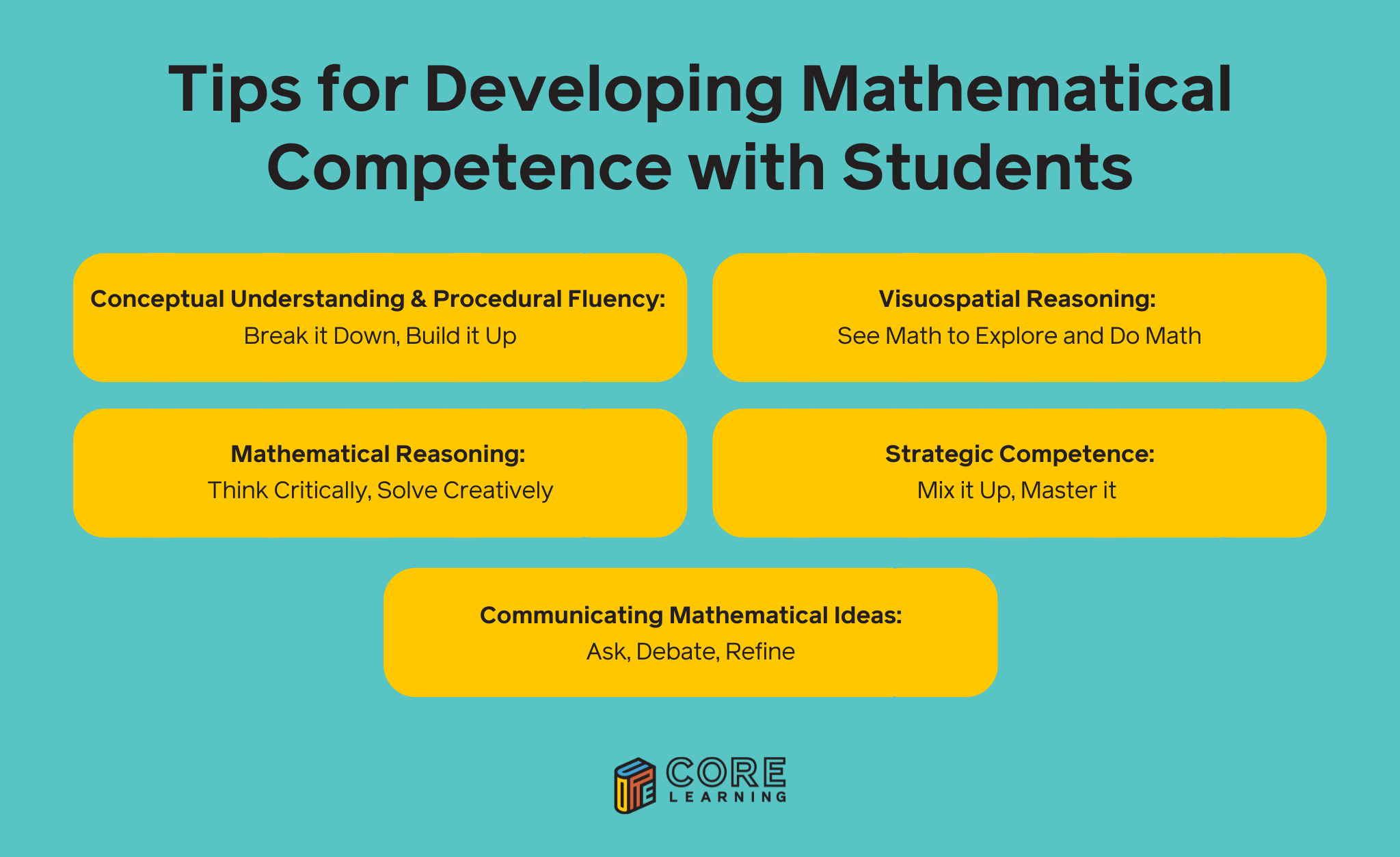
Mastering complex math isn’t a solitary climb up a rigid ladder; it’s a collaborative journey through intricate landscapes. We navigate these landscapes by constructing a dynamic path, weaving together two essential threads: conceptual understanding, the rich tapestry of interconnected ideas, and procedural fluency, the nimble tools to navigate math experiences effectively.

To support this engaging learning journey, consider using chunking. This involves presenting information in smaller, manageable pieces that students can gradually integrate into larger, more complex ideas. By guiding students to connect, process, and condense key concepts into bite-sized units, you enable them to anchor new information onto previously acquired knowledge, leading to deeper and more lasting understanding. Chunking isn’t about dividing a problem into smaller pieces; it’s about identifying meaningful connections and patterns within and between concepts. Chunking grants time to decode the language of math and understand the formulas, thereby enabling students to grasp the deeper meaning behind the symbols, equations, and graphs.

• Exploration of alternative paths: Exploring and comparing diverse solutions, like analyzing different graphs and equations to reach the same answer, strengthens existing knowledge and builds new neural pathways, mirroring the “chunking” approach of breaking down complex math concepts into manageable units for deeper understanding.
• Decode mathematical language: Don’t rush! Introduce mathematical language progressively, utilizing clear terminology, visual aids, and real-world connections. This empowers students to decipher mathematical symbols and equations, ultimately fostering a deeper comprehension of underlying concepts.

Visuospatial Reasoning: See Math to Explore and Do Math

Visuospatial reasoning bridges the gap between abstract concepts and human intuition, making mathematics more accessible and tangible. This bridge connects abstract symbols to tangible experiences through visualizations, diagrams, and manipulatives. Traversing this bridge strengthens neural pathways, leading to deeper understanding and lasting comprehension. But it’s not a one-way street.

The ability to translate mathematical concepts into various representations (equations, graphs, diagrams, and real-world scenarios) cultivates flexible mental models and robust problem-solving skills. This process reveals the underlying principles, relationships, and structures inherent in mathematics. By actively manipulating and connecting these different representations, students gain a deeper understanding of the interconnected and systematic nature of mathematical concepts.

• Visualization exercises: Guide students to visualize shapes, processes, and transformations in their minds, building mental models, and testing out intuitions.
• Manipulatives and visuals: Utilize concrete objects, visual aids, and analogies to bridge the gap between abstract concepts and tangible experiences.

Mathematical Reasoning: Think Critically, Solve Creatively

Mathematical reasoning is the art of navigating the world of numbers and ideas. It’s the ability to think critically, creatively, and flexibly while weaving together logic and ingenuity to make sense of patterns, connections, and relationships. It’s about connecting symbols and representations, exploring diverse strategies like estimation, modeling, and analogies, and ultimately solving problems in innovative ways. This multifaceted skill empowers us to unravel the beauty and intricacies of mathematics, not just through rigid logic but through a vibrant interplay of intuition, imagination, and perseverance.

• Comparative analysis: Encourage students to “zoom out” and see the bigger math picture. By comparing and contrasting concepts, students make connections and uncover the underlying structures that unify different mathematical ideas.
• Metacognitive reflection: Guide students to reflect on their thinking processes, explaining visualizations, representations, and symbol connections. This builds self-awareness and reinforces learning.

Strategic Competence: Mix it Up, Master it 

Intentional practice of familiar problems strengthens dedicated neural pathways, making them efficient for specific approaches; however, this repetition alone limits flexibility. Interleaving takes learning beyond mastering individual problems. It challenges the brain to retrieve and dynamically combine different concepts and strategies to solve ever-changing problems. Interleaving doesn’t just strengthen existing neural pathways; it forges new connections between them, weaving a richer, interconnected web of understanding. This web makes thinking more nuanced, adaptable, and capable of tackling diverse challenges with a wider range of strategies and approaches.

• Randomized problem types: Mixing diverse skills and approaches within and across sets keeps students engaged and challenges them to apply their knowledge in different contexts.
• Challenge sets with varying difficulty: Combining problems of varying complexities within sets promotes confidence, perseverance, and adaptability.

Communicating Mathematical Ideas: Ask, Debate, Refine

Mathematical communication is a multifaceted skill that leverages a diverse repertoire of skills, including precise language, symbols, diagrams, graphs, and real-world connections. Guided by critical thinking and clear reasoning, it serves to justify problems’ solutions, foster understanding, and illuminate mathematical concepts.